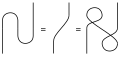Die penrosesche graphische Notation – auch als penrosesche diagrammatische Notation, Tensor-Diagramm-Notation oder auch einfach Penrose-Notation bezeichnet – ist eine von Roger Penrose vorgeschlagene Notation in der Physik und Mathematik, um eine (meist handschriftliche) visuelle Darstellung multilinearer Abbildungen oder Tensoren zu erhalten. Ein Diagramm besteht hierbei aus geschlossenen Formen, welche über Linien verbunden sind.
Die Notation wurde ausgiebig von Predrag Cvitanović erforscht, welcher diese Notation zur Einstufung klassischer Lie-Gruppen verwendet. Die Notation wurde generalisiert, um die Theorie zu Spin-Netzwerken in der Physik sowie die Präsenz von Matrix-Gruppen in der linearen Algebra darzustellen.
Multilineare Algebra
In der multilinearen Algebra entspricht jede Form einer multilinearen Funktion. Die Linien an Formen repräsentieren die Eingänge oder Ausgänge der Funktion. Die Verbindung dieser Ein- und Ausgänge entspricht der Komposition der jeweiligen Funktionen.
Tensoren
In der Tensoralgebra ist ein bestimmter Tensor als bestimmte Form dargestellt. Linien nach oben und unten abstrahieren obere und untere Indizes der jeweiligen Tensoren. Verbindungen zwischen zwei Formen entspricht der Kontraktion der Indizes. Ein Vorteil dieser Notation ist, dass man nicht neue Buchstaben für neue Indizes erfinden muss. Die Notation ist auch ausdrücklich basisunabhängig.
Beispiele
-
Vektor

-
Vektor

-
Vektor

-
Vektor

-
Tensor

-
Tensor

-
Kronecker-Delta

-
Hermitesches Skalarprodukt




(
siehe auch: Bra-Ket)
-
Metrischer Tensor

-
Metrischer Tensor

-
Symmetrisierung

-
Asymmetrisierung
![{\displaystyle E_{[a_{1}\ldots a_{n}]}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a02240bd4bb0da503477e774c24951d6fb4a3922)
-
Tensor 
-

-

-
![{\displaystyle \xi ^{a}\,\lambda _{ab[c}^{(d}\,D_{fg]}^{e)b}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/793f74d267912d285fb58db9d6f1254547727a3b)
(mit

)
-
-

-
Fakultät

-
Determinante 
-


-

(Inverse der

-Matrix

)
-
Spurfunktion

-
Strukturkonstante 
-
![{\displaystyle \gamma _{[\alpha \beta }^{\xi }\,\gamma _{\chi ]\xi }^{\zeta }=0}](https://wikimedia.org/api/rest_v1/media/math/render/svg/19699acb511e38dfe0e84b47ee7276be771763f5)
-
![{\displaystyle \gamma _{[\alpha \beta }^{\xi }\,\gamma _{\chi ]\xi }^{\zeta }}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5aaf60dfe7324def1a0628552dbc87e9061acf10)




-
Killing-Form 


-
Kovariante Ableitung ![{\displaystyle 12\nabla _{\mu }\left\{\xi ^{a}\lambda _{ab[c}^{(d}D_{fg]}^{e)b}\right\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c5fb11ce3222b3d59a55484c91ec0af501e740f2)
-
-
Ricci-Identität


-
Ricci-Tensor 
-
Antisymmetrie des riemannschen Krümmungstensors

-
Bianchi-Symmetrie ![{\displaystyle R_{[abc]}^{\ \ \ d}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d938019c0394c58397459d7c9ab5b5cfebd98183)




-
Bianchi-Identität ![{\displaystyle \nabla _{[a}R_{bc]d}^{\ \ \ e}=0}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3896d2dbc992145ed836177f508a50380f70d023)
-

(z. B.

)
-

-

Siehe auch
Literatur
- Roger Penrose: The Road to Reality: A Complete Guide to the Laws of the Universe. Jonathan Cape, London u. a. 2004, ISBN 0-224-04447-8.
- Roger Penrose, Wolfgang Rindler: Spinors and Space-Time: Vol I, Two-Spinor Calculus and Relativistic Fields. Cambridge University Press, 1984, ISBN 0-521-24527-3.
- Roger Penrose, Wolfgang Rindler: Spinors and Space-Time: Vol. II, Spinor and Twistor Methods in Space-Time Geometry. Cambridge University Press, 1986, ISBN 0-521-25267-9.
Weblinks
Commons: Penrose graphical notation – Sammlung von Bildern, Videos und Audiodateien
 Vektor
Vektor Vektor
Vektor Vektor
Vektor Vektor
Vektor Tensor
Tensor Tensor
Tensor Kronecker-Delta
Kronecker-Delta Hermitesches Skalarprodukt
Hermitesches Skalarprodukt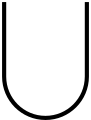 Metrischer Tensor
Metrischer Tensor Metrischer Tensor
Metrischer Tensor Symmetrisierung
Symmetrisierung Asymmetrisierung
Asymmetrisierung





 Fakultät
Fakultät


 Spurfunktion
Spurfunktion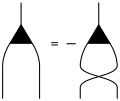





 Ricci-Identität
Ricci-Identität
 Antisymmetrie des riemannschen Krümmungstensors
Antisymmetrie des riemannschen Krümmungstensors



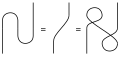


























![{\displaystyle E_{[a_{1}\ldots a_{n}]}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a02240bd4bb0da503477e774c24951d6fb4a3922)







![{\displaystyle \xi ^{a}\,\lambda _{ab[c}^{(d}\,D_{fg]}^{e)b}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/793f74d267912d285fb58db9d6f1254547727a3b)





















![{\displaystyle \gamma _{[\alpha \beta }^{\xi }\,\gamma _{\chi ]\xi }^{\zeta }=0}](https://wikimedia.org/api/rest_v1/media/math/render/svg/19699acb511e38dfe0e84b47ee7276be771763f5)

![{\displaystyle \gamma _{[\alpha \beta }^{\xi }\,\gamma _{\chi ]\xi }^{\zeta }}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5aaf60dfe7324def1a0628552dbc87e9061acf10)









![{\displaystyle 12\nabla _{\mu }\left\{\xi ^{a}\lambda _{ab[c}^{(d}D_{fg]}^{e)b}\right\}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c5fb11ce3222b3d59a55484c91ec0af501e740f2)










![{\displaystyle R_{[abc]}^{\ \ \ d}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d938019c0394c58397459d7c9ab5b5cfebd98183)




![{\displaystyle \nabla _{[a}R_{bc]d}^{\ \ \ e}=0}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3896d2dbc992145ed836177f508a50380f70d023)